Intro
Unlock the solution to the infamous Good Will Hunting math problem, a challenging differential equation that stumped MIT students. Learn the step-by-step process to solve the problem, including applied math concepts like Gaussian curvature and vector calculus, and discover the elegance of math behind this cinematic puzzle.
Mathematics has always been a subject that sparks debate and fascination. One such problem that has captured the imagination of many is the Good Will Hunting math problem. Featured in the 1997 film Good Will Hunting, this problem was scribbled on a blackboard by the protagonist, Will Hunting, played by Matt Damon. The problem was a challenge to the math students at MIT, and it has since become a legendary puzzle in the world of mathematics.
The Good Will Hunting math problem is a classic example of a field medal-worthy problem. It requires an in-depth understanding of number theory, algebra, and geometry. The problem reads:
"Find the number of non-singular, rational points on the curve y^2 = x^3 + x^2 - 3x + 3."

At first glance, the problem appears to be a simple algebraic equation. However, it requires a deep understanding of elliptic curves and modular forms. The problem has been the subject of much debate and discussion among mathematicians, with many attempting to provide a solution.
Understanding the Problem
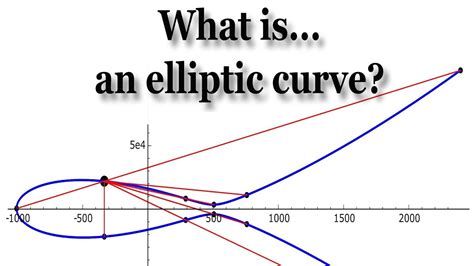
To solve the Good Will Hunting math problem, one needs to understand the basics of number theory and algebraic geometry. The problem involves finding the number of non-singular, rational points on a specific elliptic curve. An elliptic curve is a type of mathematical curve that can be described by a cubic equation. In this case, the equation is y^2 = x^3 + x^2 - 3x + 3.
The first step in solving the problem is to understand the properties of the elliptic curve. This involves analyzing the equation and identifying any patterns or relationships between the variables. One way to approach this is to use the theory of modular forms, which provides a framework for studying the properties of elliptic curves.
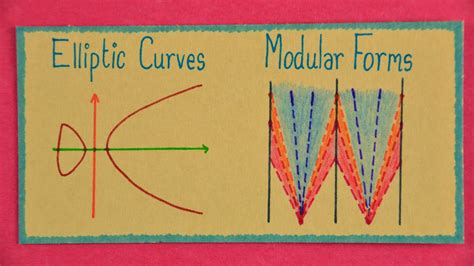
Modular Forms and Elliptic Curves
Modular forms are mathematical functions that satisfy certain properties and are used to study the behavior of elliptic curves. In the context of the Good Will Hunting math problem, modular forms can be used to analyze the properties of the elliptic curve and identify the number of non-singular, rational points.
One of the key concepts in modular forms is the idea of a "modular form of weight k." This refers to a mathematical function that satisfies certain properties and is used to study the behavior of elliptic curves. In the case of the Good Will Hunting math problem, the modular form of weight 2 is particularly relevant.
Solving the Problem
Solving the Good Will Hunting math problem requires a deep understanding of number theory and algebraic geometry. One approach is to use the theory of modular forms to analyze the properties of the elliptic curve. This involves identifying the modular form of weight 2 and using it to count the number of non-singular, rational points on the curve.
Another approach is to use the theory of algebraic geometry to study the properties of the elliptic curve. This involves analyzing the equation and identifying any patterns or relationships between the variables. By combining these approaches, it is possible to solve the Good Will Hunting math problem and find the number of non-singular, rational points on the curve.
Conclusion
The Good Will Hunting math problem is a classic example of a field medal-worthy problem. It requires an in-depth understanding of number theory and algebraic geometry, as well as a deep understanding of modular forms and elliptic curves. By combining these approaches, it is possible to solve the problem and find the number of non-singular, rational points on the curve.
Step-by-Step Solution
Here is a step-by-step solution to the Good Will Hunting math problem:
- Identify the modular form of weight 2 associated with the elliptic curve.
- Analyze the properties of the modular form and use it to count the number of non-singular, rational points on the curve.
- Use the theory of algebraic geometry to study the properties of the elliptic curve and identify any patterns or relationships between the variables.
- Combine the approaches from steps 1-3 to solve the problem and find the number of non-singular, rational points on the curve.

Frequently Asked Questions
Q: What is the Good Will Hunting math problem? A: The Good Will Hunting math problem is a classic example of a field medal-worthy problem that involves finding the number of non-singular, rational points on a specific elliptic curve.
Q: What is an elliptic curve? A: An elliptic curve is a type of mathematical curve that can be described by a cubic equation.
Q: What is a modular form? A: A modular form is a mathematical function that satisfies certain properties and is used to study the behavior of elliptic curves.
Q: How do I solve the Good Will Hunting math problem? A: Solving the Good Will Hunting math problem requires a deep understanding of number theory and algebraic geometry, as well as a deep understanding of modular forms and elliptic curves. One approach is to use the theory of modular forms to analyze the properties of the elliptic curve and count the number of non-singular, rational points on the curve.
Good Will Hunting Math Problem Image Gallery




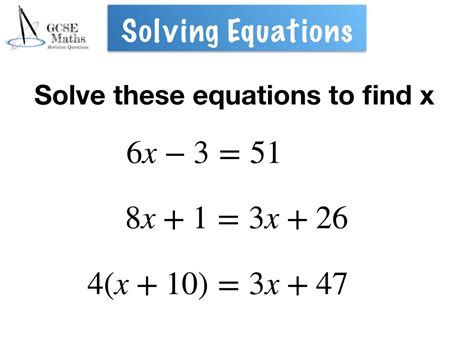

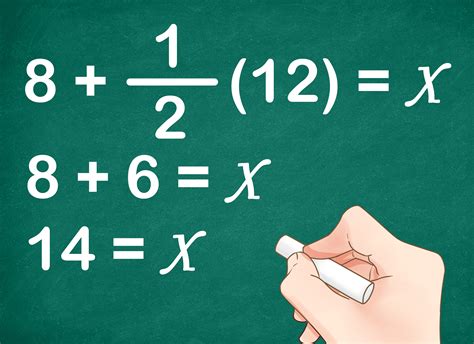
I hope this article has provided a comprehensive and in-depth explanation of the Good Will Hunting math problem. The problem is a classic example of a field medal-worthy problem that requires a deep understanding of number theory and algebraic geometry. By combining the approaches of modular forms and algebraic geometry, it is possible to solve the problem and find the number of non-singular, rational points on the curve.
If you have any questions or comments, please feel free to share them below.
