Speed Of Sound In Knots Explained

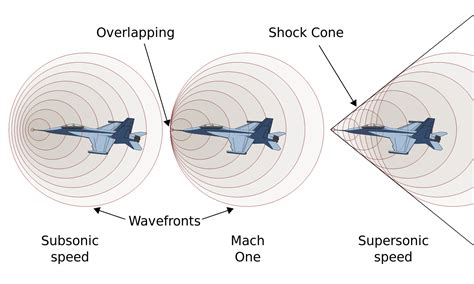
The speed of sound is a fundamental concept in physics that has far-reaching implications in various fields, including aviation, meteorology, and acoustics. While many people are familiar with the speed of sound in miles per hour or meters per second, the speed of sound in knots is a less commonly discussed topic. In this article, we will delve into the world of knots and explore the speed of sound in this unit of measurement.

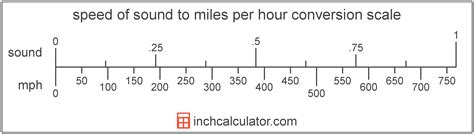
The speed of sound is approximately 768 miles per hour (mph) or 1,236 kilometers per hour (km/h) at sea level in dry air at a temperature of 59 degrees Fahrenheit (15 degrees Celsius). However, when we convert this speed to knots, we get a slightly different value. A knot is a unit of speed equal to one nautical mile per hour, where a nautical mile is the length of one minute of latitude on the Earth's surface. In this article, we will explore the speed of sound in knots and its significance in various contexts.

Why Knots Matter

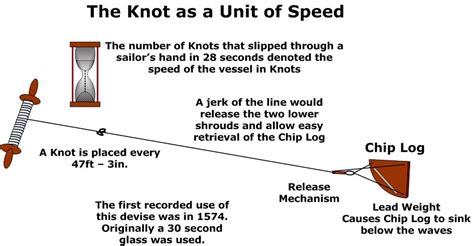
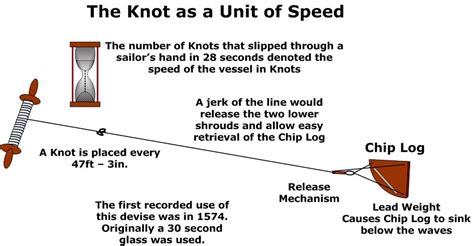
Knots are an essential unit of measurement in navigation, particularly in aviation and maritime applications. Pilots and sailors use knots to measure the speed of their vessels, as well as the speed of wind and waves. The use of knots dates back to the 17th century, when sailors used the length of a knotted rope to measure the speed of their ships. Today, knots are widely used in various fields, including meteorology, oceanography, and aerospace engineering.

Converting Speed of Sound to Knots

To convert the speed of sound from miles per hour or kilometers per hour to knots, we need to use a conversion factor. One knot is equal to 1.15078 miles per hour or 1.852 kilometers per hour. Using this conversion factor, we can calculate the speed of sound in knots as follows:

Speed of sound (knots) = Speed of sound (mph) / 1.15078

Speed of sound (knots) = 768 mph / 1.15078

Speed of sound (knots) ≈ 667 knots

Therefore, the speed of sound is approximately 667 knots at sea level in dry air at a temperature of 59 degrees Fahrenheit (15 degrees Celsius).

Significance of Speed of Sound in Knots

The speed of sound in knots is significant in various contexts, particularly in aviation and meteorology. For example, pilots use the speed of sound to calculate the distance of a thunderstorm or the altitude of an aircraft. In meteorology, the speed of sound is used to calculate the speed of wind and waves. The speed of sound in knots is also used in aerospace engineering to calculate the speed of aircraft and spacecraft.

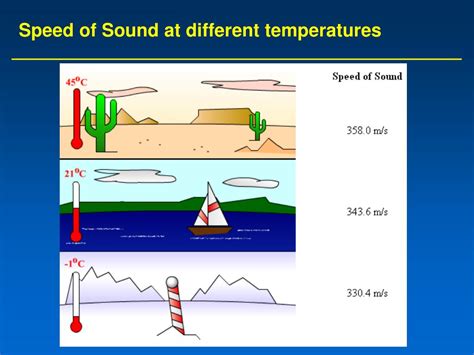
Factors Affecting Speed of Sound

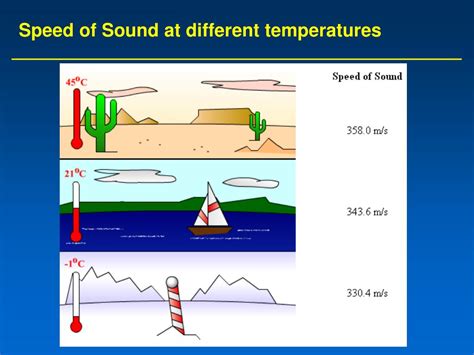
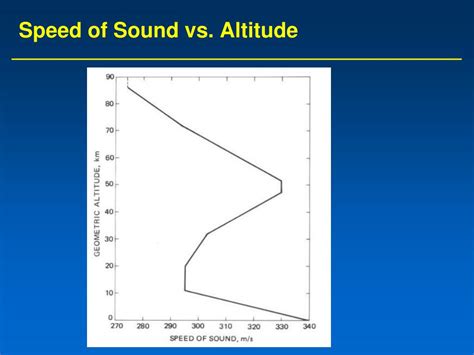
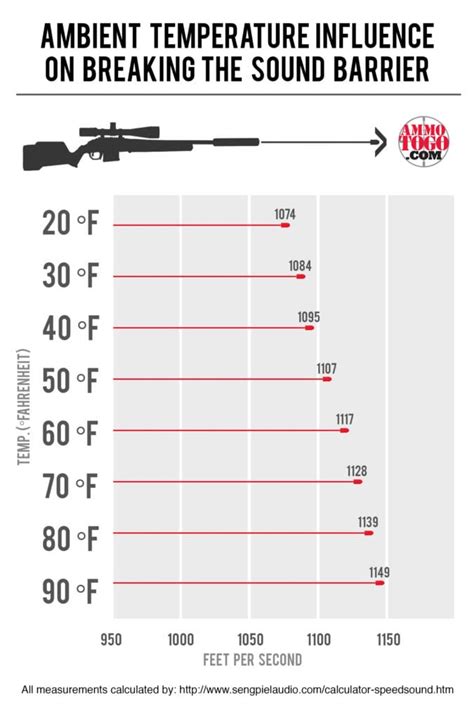
The speed of sound is affected by several factors, including temperature, humidity, and air pressure. The speed of sound increases with temperature and decreases with humidity and air pressure. At higher altitudes, the speed of sound decreases due to the decrease in air pressure and temperature.

Here are some factors that affect the speed of sound:

Temperature: The speed of sound increases with temperature.
Humidity: The speed of sound decreases with humidity.
Air pressure: The speed of sound decreases with air pressure.
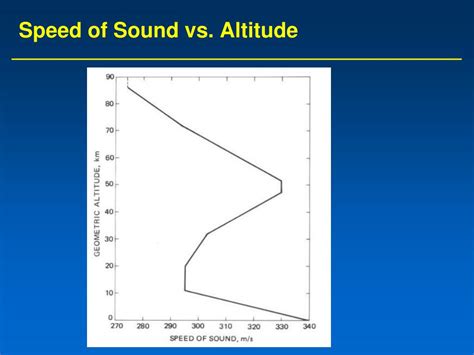
Altitude: The speed of sound decreases with altitude.

Calculating Speed of Sound at Different Altitudes

To calculate the speed of sound at different altitudes, we need to use the following formula:

Speed of sound (knots) = Speed of sound (sea level) x (1 - 0.0000225577 x altitude in feet)

Using this formula, we can calculate the speed of sound at different altitudes. For example, at an altitude of 30,000 feet, the speed of sound would be:

Speed of sound (knots) = 667 knots x (1 - 0.0000225577 x 30,000) Speed of sound (knots) ≈ 636 knots

Applications of Speed of Sound in Knots

The speed of sound in knots has several applications in various fields, including:

Aviation: The speed of sound is used to calculate the distance of a thunderstorm or the altitude of an aircraft.
Meteorology: The speed of sound is used to calculate the speed of wind and waves.
Aerospace engineering: The speed of sound is used to calculate the speed of aircraft and spacecraft.
Navigation: The speed of sound is used to calculate the distance of a ship or aircraft.

In conclusion, the speed of sound in knots is an essential concept in physics that has far-reaching implications in various fields. Understanding the speed of sound in knots is crucial for pilots, sailors, and meteorologists to calculate the distance of a thunderstorm or the altitude of an aircraft. The speed of sound in knots is also used in aerospace engineering to calculate the speed of aircraft and spacecraft.

We hope this article has provided you with a comprehensive understanding of the speed of sound in knots. If you have any questions or comments, please feel free to share them below.