5 Infinite Solution Examples

The concept of infinite solutions has been a topic of interest in various fields, including mathematics, physics, and philosophy. It refers to the idea that a problem or equation can have an infinite number of solutions, each with its own unique characteristics. In this article, we will explore five examples of infinite solutions, highlighting their significance and implications.

Infinite solutions have far-reaching consequences, as they challenge our traditional understanding of problem-solving and the nature of reality. By examining these examples, we can gain insights into the complexities of the universe and the human experience. Whether you are a mathematician, scientist, or simply a curious individual, the concept of infinite solutions is sure to fascinate and inspire.

The study of infinite solutions has led to numerous breakthroughs in various fields, from calculus to quantum mechanics. It has also raised fundamental questions about the nature of reality, free will, and the human condition. As we delve into these examples, we will see how infinite solutions can help us better understand the world and our place within it.

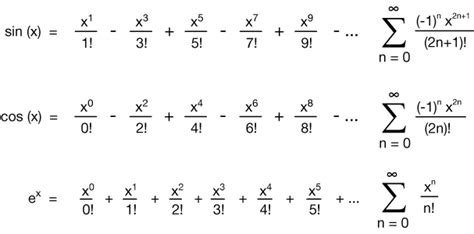
Infinite Series

Infinite series are a classic example of infinite solutions. An infinite series is a sum of an infinite number of terms, where each term is defined by a specific formula. For instance, the geometric series 1 + x + x^2 + x^3 + ... has an infinite number of terms, each depending on the value of x. The sum of this series can be calculated using the formula 1 / (1 - x), which is valid for |x| < 1.

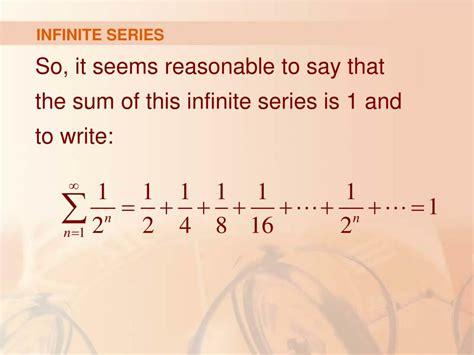
Convergence and Divergence

The convergence or divergence of an infinite series depends on the values of its terms. If the terms decrease rapidly enough, the series may converge to a finite sum. However, if the terms do not decrease sufficiently, the series may diverge, resulting in an infinite sum. Understanding the behavior of infinite series is crucial in mathematics and physics, as it helps us model real-world phenomena, such as population growth, chemical reactions, and electrical circuits.

Fractals and Self-Similarity

Fractals are geometric shapes that exhibit self-similarity, meaning they appear the same at different scales. The Sierpinski triangle, the Mandelbrot set, and the Julia set are famous examples of fractals. These shapes have infinite solutions, as they can be divided into smaller copies of themselves, repeating the same pattern infinitely. Fractals have numerous applications in physics, biology, and computer science, including modeling coastlines, trees, and galaxy distributions.

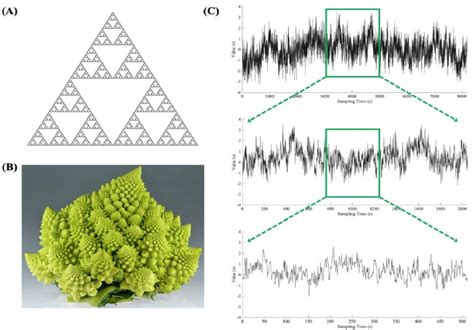
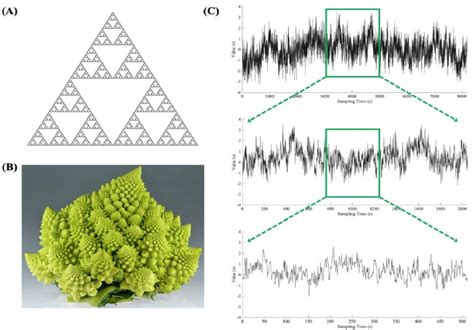
Fractal Dimensions

Fractals often have non-integer dimensions, which can be calculated using various methods, such as the box-counting method or the correlation dimension. The fractal dimension of a shape can provide insights into its properties, such as its surface area, volume, and scaling behavior. Understanding fractals and their dimensions is essential in fields like materials science, where it helps us design new materials with unique properties.

Quantum Mechanics and Wave Functions

In quantum mechanics, wave functions describe the behavior of particles, such as electrons and photons. These wave functions can have infinite solutions, as they satisfy the Schrödinger equation, a partial differential equation that governs the time-evolution of quantum systems. The solutions to the Schrödinger equation are wave functions, which can be combined to form a complete set of basis states.

Wave Function Collapse

When a measurement is made on a quantum system, the wave function collapses to one of the possible outcomes, a process known as wave function collapse. This collapse is a fundamental aspect of quantum mechanics, as it introduces an element of randomness and uncertainty into the behavior of particles. Understanding wave function collapse is crucial in fields like quantum computing, where it helps us develop new algorithms and technologies.

Calculus and Infinite Limits

In calculus, infinite limits are used to describe the behavior of functions as the input variable approaches infinity. For example, the limit of the function 1/x as x approaches infinity is 0, indicating that the function decreases without bound as x increases. Infinite limits are essential in calculus, as they help us model real-world phenomena, such as population growth, chemical reactions, and electrical circuits.

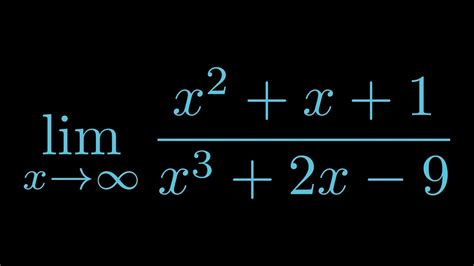
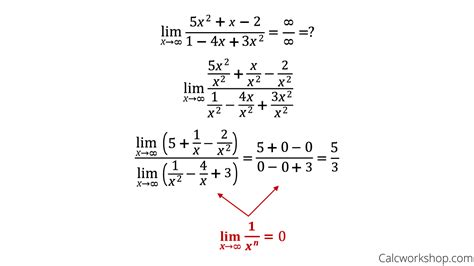
Improper Integrals

Improper integrals are integrals that have infinite limits of integration or integrands that become infinite at certain points. These integrals can be evaluated using various techniques, such as substitution, integration by parts, or contour integration. Understanding improper integrals is crucial in fields like physics and engineering, where they help us model real-world phenomena, such as electromagnetic radiation, heat transfer, and fluid dynamics.

Number Theory and Infinite Primes

In number theory, infinite primes refer to the infinite number of prime numbers that exist. Prime numbers are positive integers that are divisible only by themselves and 1. The distribution of prime numbers is a fundamental problem in number theory, and understanding infinite primes is essential in fields like cryptography, where they help us develop secure encryption algorithms.

Prime Number Theorem

The prime number theorem describes the distribution of prime numbers among the positive integers. It states that the number of prime numbers less than or equal to x grows like x / ln(x) as x approaches infinity. Understanding the prime number theorem is crucial in fields like computer science, where it helps us develop efficient algorithms for primality testing and factorization.

Infinite Solution Image Gallery

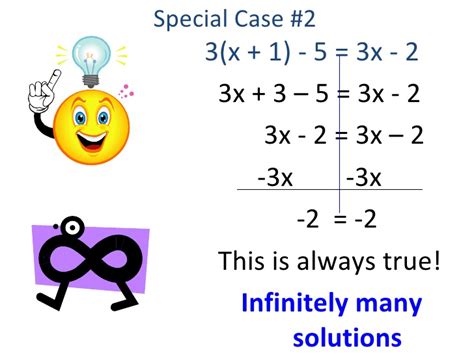
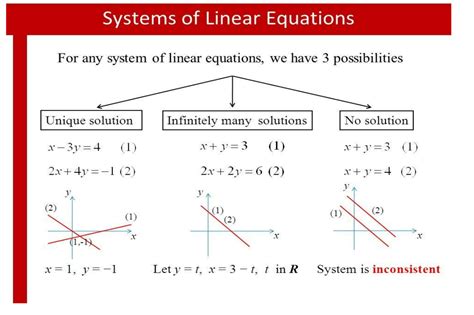
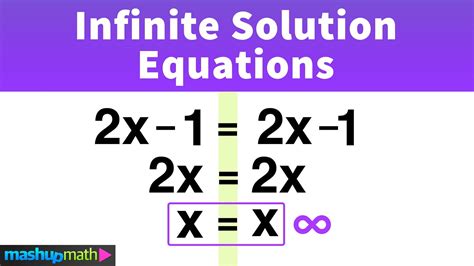
In conclusion, infinite solutions are a fascinating and complex topic that has far-reaching implications in various fields. By exploring these examples, we can gain a deeper understanding of the nature of reality, the behavior of particles, and the human experience. Whether you are a mathematician, scientist, or simply a curious individual, the concept of infinite solutions is sure to inspire and challenge your thinking. We invite you to share your thoughts and insights on this topic, and to continue exploring the many wonders of infinite solutions. Please comment below, share this article with others, or take a moment to reflect on the infinite possibilities that surround us.